Addition and subtraction of algebraic fractions

$Addition and subtraction of algebraic fractions$

Addition and subtraction of algebraic fractions

In case of addition and subtraction of algebraic fractions with a common denominator, we should simply add or subtract the numerators. Then, the sum is reduced to its lowest terms. For example:

********************

10 Math Problems officially announces the release of Quick Math Solver and 10 Math Problems, Apps on Google Play Store for students around the world.

Install Quick Math Solver

********************

Install 10 Math Problems

********************

$Example of addition and subtraction of algebraic fractions$

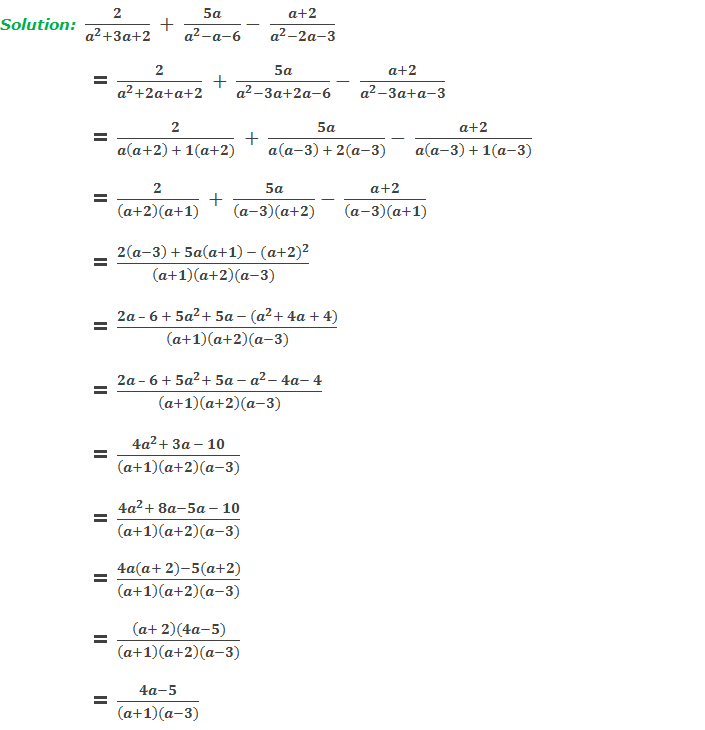
In the case of addition and subtraction of the algebraic fractions with different denominator, we follow the following steps:

1. Find the LCM of denominators.

2. Divide the LCM by each denominator and multiply the quotient so obtained by the corresponding numerator.

3. Carry out the operation of addition or subtraction in the numerator.

4. Reduce the sum to its lowest terms.

Look at the following worked out examples:

Workout Examples

Example 1: Simplify: (i) x/4a+ x/4a (ii) x^2/(x^2-y^2 ) + y^2/(x^2-y^2 ) - 2xy/(x^2-y^2 )

Example 2: Simplify: (i) 1/(x+1)+ 1/(x-1) (ii) (x+2)/(x-2) - (x-2)/(x+2)

Solution: 2(ii) (x+2)/(x-2) - (x-2)/(x+2) = (〖(x+2)〗^2- 〖(x-2)〗^2)/((x-2)(x+2)) = (x^2 + 2.x2 +〖 2〗^2 - (x^2 - 2.x.2 +〖 2〗^2))/((x+2)(x-2)) = (x^2 + 4x + 4 - x^2+ 4x - 4)/(x^2-2^2 ) = 8x/(x^2-4)

Example 3: Simplify: (i) (x-2)/(x+2)- (x-2)/(x^2-4) (ii) 2/(x+1) + 2x/(x-1) - (x^2+3)/(x^2-1)

Solution: 3(i) (x-2)/(x+2) - (x-2)/(x^2-4) = (x-2)/(x+2) - (x-2)/(x^2-2^2 ) = (x-2)/(x+2)- (x-2)/((x+2)(x-2)) = (x-2)/(x+2)- 1/(x+2) = (x-2-1)/(x+2) = (x-3)/(x+2)

Solution: 3(ii) 2/(x+1) + 2x/(x-1) - (x^2+3)/(x^2-1) = 2/(x+1) + 2x/(x-1) - (x^2+3)/((x+1)(x-1)) = (2(x-1) + 2x(x+1) - (x^2+3))/((x+1)(x-1)) = (2x – 2 + 2x^2+ 2x - x^2-3)/((x+1)(x-1)) = (x^2+ 4x - 5)/((x+1)(x-1)) = (x^2+ 5x - x - 5)/((x+1)(x-1)) = (x(x + 5) - 1(x + 5))/((x+1)(x-1)) = ((x + 5)(x -1))/((x + 1)(x -1)) = (x + 5)/(x + 1)

Example 4: Simplify: (i) 1/(a^2-3a+2) + 2/(a^2-4a+3) (ii) (x+2)/(x^2+x) - 3/(x^2-x-2)

Solution: 4(i) 1/(a^2-3a+2) + 2/(a^2-4a+3) = 1/(a^2-2a-a+2) + 2/(a^2-3a-a+3) = 1/(a(a-2)-1(a-2)) + 2/(a(a-3)-1(a-3)) = 1/((a-2)(a-1)) + 2/((a-3)(a-1)) = (1(a-3)+2(a-2))/((a-1)(a-2)(a-3)) = (a-3+2a-4)/((a-1)(a-2)(a-3)) = (3a-7)/((a-1)(a-2)(a-3))

Solution: 4(ii) (x+2)/(x^2+x) - 3/(x^2-x-2) = (x+2)/(x(x+1)) - 3/(x^2-2x+x-2) = (x+2)/(x(x+1)) - 3/(x(x-2)+1(x-2)) = (x+2)/(x(x+1)) - 3/((x-2)(x+1)) = ((x+2)(x-2)-3x)/(x(x+1)(x-2)) = (x^2-2^2-3x)/(x(x+1)(x-2)) = (x^2-4-3x)/(x(x+1)(x-2)) = (x^2-3x-4)/(x(x+1)(x-2)) = (x^2-4x+x-4)/(x(x+1)(x-2)) = (x(x-4)+1(x-4))/(x(x+1)(x-2)) = ((x-4)(x+1))/(x(x+1)(x-2)) = (x-4)/(x(x-2))

Example 5: Simplify: 2/(a^2+3a+2) + 5a/(a^2-a-6)- (a+2)/(a^2-2a-3)

Solution: (5) 2/(a^2+3a+2) + 5a/(a^2-a-6)- (a+2)/(a^2-2a-3) = 2/(a^2+2a+a+2) + 5a/(a^2-3a+2a-6)- (a+2)/(a^2-3a+a-3) = 2/(a(a+2) + 1(a+2)) + 5a/(a(a-3) + 2(a-3))- (a+2)/(a(a-3) + 1(a-3)) = 2/((a+2)(a+1)) + 5a/((a-3)(a+2))- (a+2)/((a-3)(a+1)) = (2(a-3) + 5a(a+1) -〖 (a+2)〗^2)/((a+1)(a+2)(a-3)) = (2a – 6 + 5a^2+ 5a - (a^2+ 4a + 4))/((a+1)(a+2)(a-3)) = (2a – 6 + 5a^2+ 5a - a^2- 4a- 4)/((a+1)(a+2)(a-3)) = (4a^2+ 3a - 10)/((a+1)(a+2)(a-3)) = (4a^2+ 8a-5a - 10)/((a+1)(a+2)(a-3)) = (4a(a+ 2)-5(a+2))/((a+1)(a+2)(a-3)) = ((a+ 2)(4a-5))/((a+1)(a+2)(a-3)) = (4a-5)/((a+1)(a-3))

You can comment your questions or problems regarding addition and subtraction of algebraic fractions here.

About author: Min Rajbanshi

I am a school math teacher and a blogger since 2012. I do blogging on a variety of topics including math. Over the years, I've explored and written about a wide range of topics, with a particular emphasis on mathematics. My passion for education extends beyond the classroom, as I enjoy sharing knowledge and insights on various subjects through my blog.

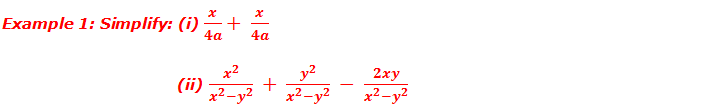
0 comments:

Subscribe to: Post Comments (Atom)