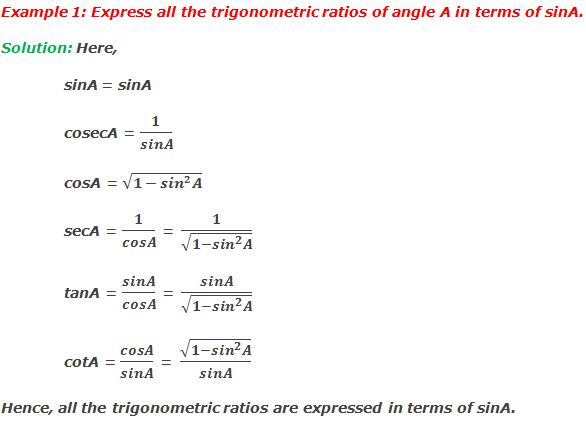
Conversion of Trigonometric Ratios

0 comments

Conversion of Trigonometric Ratios

Conversion of Trigonometric Ratios

There are six trigonometric ratios. If one of them is known, the remaining five can be obtained by the conversion of trigonometric ratios. If we know any one of the trigonometric ratio, then the rest can be converted in terms of that known ratio. This can be done by using either of the following two methods:

(a) Using basic trigonometric relations

(b) Using Pythagoras' theorem



********************


10 Math Problems officially announces the release of Quick Math Solver and 10 Math Problems, Apps on Google Play Store for students around the world.


Install Quick Math Solver

********************


Install 10 Math Problems


********************



 

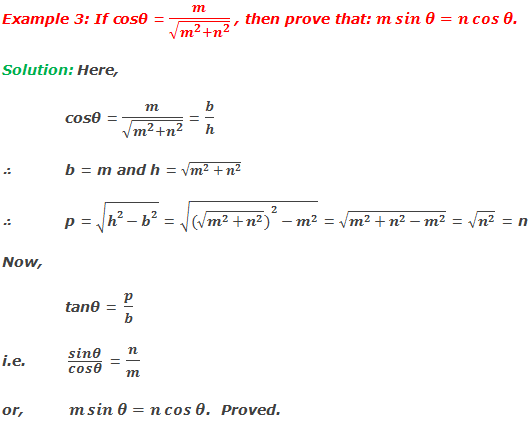
Lets study the following worked out examples:


Example 1: Express all the trigonometric ratios of angle A in terms of sinA. Solution: Here, sinA = sinA cosecA = 1/sinA cosA = √(1-〖sin〗^2 A) secA = 1/cosA = 1/√(1-〖sin〗^2 A) tanA = sinA/cosA = sinA/√(1-〖sin〗^2 A) cotA = cosA/sinA = √(1-〖sin〗^2 A)/sinA Hence, all the trigonometric ratios are expressed in terms of sinA.

Example 2: If Sinθ = 12/13, find cosθ and tanθ. Solution: Here, Sinθ = 12/13 = p/h ∴ p = 12 and h = 13 ∴ b = √(h^2-p^2 ) = √(〖13〗^2-〖12〗^2 ) = √(169-144) = √25 = 5 ∴ cosθ = b/h = 5/13 And, tanθ = p/b = 12/5


Example 3: If cosθ = m/√(m^2+n^2 ) , then prove that: m sin⁡〖θ=n cos⁡θ 〗. Solution: Here, cosθ = m/√(m^2+n^2 ) = b/h ∴ b = m and h = √(m^2+n^2 ) ∴ p = √(h^2-b^2 ) = √(〖(√(m^2+n^2 ))〗^2-m^2 ) = √(m^2+n^2-m^2 ) = √(n^2 ) = n Now, tanθ = p/b i.e. sin⁡θ/cos⁡θ = n/m or, m sin⁡〖θ=n cos⁡θ 〗. Proved.

Example 4: If 3Sinθ + 4cosθ = 5, show that cosθ = 4/5.

Solution: Here,

          3sinθ + 4cosθ = 5

or,     5 – 4cosθ = 3sinθ

          Squaring both sides, we get

          25 – 40cosθ + 16cos2θ = 9sin2θ

or,     16cos2θ – 40cosθ + 25 = 9(1 – cos2θ)

or,     16cos2θ – 40cosθ + 25 = 9 – 9cos2θ

or,     25cos2θ – 40cosθ + 16 = 0

or,     (5cosθ – 4)2 = 0

or,     5cosθ – 4 = 0

or,     5cosθ = 4

or,     cosθ = 4/5. Shown.


Example 5: If tanA = 1/2 and tanB = 1/3 , then show that cosA.sinB + sinA.cosB = 1/√2. Solution: Here, tanA = 1/2 = p/b ∴ p = 1 and b = 2 ∴ h = √(p^2+b^2 ) = √(1^2+2^2 ) = √(1+4) = √5 ∴ sinA = p/h = 1/√5 and cosA = b/h = 2/√5 Again, tanB = 1/3 = p/b ∴ p = 1 and b = 3 ∴ h = √(p^2+b^2 ) = √(1^2+3^2 ) = √(1+9) = √10 ∴ sinB = p/h = 1/√10 and cosB = b/h = 3/√10 Now, cosA.sinB + sinA.cosB = 2/√5 . 1/√10+1/√5 . 3/√10 = 2/(√5 √10) + 3/(√5 √10) = (2+3)/(√5 √10) = 5/(√5 √(5×2)) = (√5 .√5)/(√5 √5.√2) = 1/√2 proved.

Example 6: If sinα = cosβ = √3/2 , then show that (tan⁡α -〖 tan〗⁡β)/(1 +〖 tan〗⁡α tan⁡β )=1/√3 . Solution: Here, sinα = √3/2 ∴ cosα = √(1-〖sin〗^2 α) = √(1- (√3/2)^2 ) = √(1- 3/4) = √(1/4) = 1/2 ∴ tanα = sinα/cosα = (√3/2 )/(1/2) = √3 Again, cosβ = √3/2 ∴ sinβ = √(1-〖cos〗^2 β) = √(1- (√3/2)^2 ) = √(1- 3/4) = √(1/4) = 1/2 ∴ tanβ = sinβ/cosβ = (1/2 )/(√3/2) = 1/√3 Now, (tan⁡α -〖 tan〗⁡β)/(1 +〖 tan〗⁡α tan⁡β ) = (√3 - 1/√3)/(1 + √3 . 1/√3) = ((3 - 1)/√3)/(1 + 1) = (2/√3)/2 = 1/√3 proved.

 

Example 7: If tanA + cotA = 3, prove that tan2A + cot2A = 7.

Solution: Here,

          tanA + cotA = 3

          Squaring both sides, we get

          tan2A + 2tanA.cotA + cot2A = 9

or,     tan2A + 2×1 + cot2A = 9   [∵ tanA × cotA = 1]

or,     tan2A + 2 + cot2A = 9

or,     tan2A + cot2A = 9 – 2

or,     tan2A + cot2A = 7.  Proved.

 

You can comment your questions or problems regarding the conversion of trigonometric ratios here.

Labels:

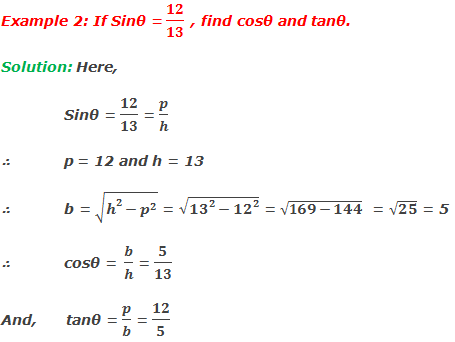
0 comments:

Subscribe to: Post Comments (Atom)