Transformation: Translation

The Translation is a transformation in which each point of the given object is displaced through definite distance and direction. The displacement is defined by a translation vector (a, b).

********************

10 Math Problems officially announces the release of Quick Math Solver and 10 Math Problems, Apps on Google Play Store for students around the world.

********************

********************

The following are the properties of translation:

1.   The object and the image under the translation are congruent.

2.   The lines joining any point of the object with its corresponding image are parallel and equal.

Example 1: Find the image of ΔABC under the translation through translation vector PQ.

Solution:

To get the image of ΔABC under the translation vector PQ draw the lines through A, B, and C which are parallel and equal to PQ in the same direction to get the images of A, B, and C and join them to get ΔA’B’C’.

 

Translation Using Co-ordinates

The image of the geometrical figures under the translation through certain translation vector can be obtained with the help of co-ordinates.

Translation Through Translation Vector T = (a, b)

Let A(2, 1) be a point and T = (2, 3) be the translation vector. To translate A(2, 1) through translation vector T = (2, 3), it has to move 2 units right and then 3 units up which is the point (4, 4) as shown in the graph given below.

Hence, A’(4, 4) is the image of the point A(2, 1) under the translation vector T = AA’ = (2, 3).

Let us see the following table of some other points on the same graph and their corresponding images under the translation through translation vector T = (2, 3).

From the above table, we can see that the image of any point under the translation through translation vector (a, b) is obtained by adding a to x-coordinate and b to y-coordinate of the given point.

Worked Out Examples

 

Example 2: If A(-4, -4), B(-2, -1) and C(-1, -5) are the vertices of a triangle ABC. Find the co-ordinates of the image of ΔABC under the translation T =(6, 5). Draw ΔABC and its image on the same graph paper.

Solution:

As A(-4, -4), B(-2, -1) and C(-1, -5) are the vertices of ΔABC, the co-ordinates of the vertices of image of ΔABC can be obtained by using the formula as below:

Drawing ΔABC and ΔA’B’C’ on the same graph paper we have figure as shown.

 

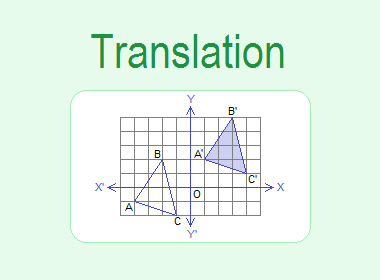
Example 3: A(-4, 2), B(0, 1) and C(-2, -3) are the vertices of ΔABC. If A’(-1, 6) be the image under the translation of vertex A. Find the images B’ and C’ of B and C under the same translation.

Solution:

Here, as the image of A(-4, 2) under the translation is A’(-1, 6),

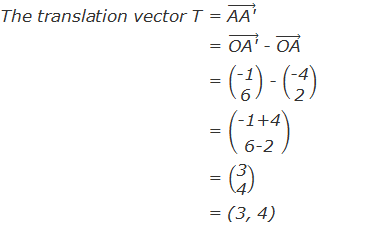
Now, under the same translation, the image of B(0, 1) is B’(0+3, 1+4) i.e. B’(3, 5) and the image of C(-2, -3) is C’(-2+3, -3+4) i.e. C’(1, 1).

Example 4: Translate a point A(2, 7) under translation T₁ = (4, -3) to the point A’. Translate A’ to A’’ under another translation T₂ =(2, 4). Find the translation vector which translates A to A’’.

Solution:

Now, let T be the translation vector which translates A(2, 7) to A’’(8, 8).

Example 5: Translate the point M(4, -5) by the translation vector T = (-3, 4) and find the image point M’ and write down the translation vector which maps M’ to M.

Solution:

Let T’ = (a, b) be the translation vector which maps M’(1, -1) back to M(4, -5). Then,

Therefore,

1+a = 4

or, a = 4 – 1

or, a = 3

And,

-1+b = -5

or, b = -5 + 1

or, b = -4

∴ The required translation vector is (3, -4) which is called the inverse of T and written as T^-1.

i.e.    If T = (a, b) then T^-1 = (-a, -b).

 

Do you have any questions regarding the translation?

You can ask your questions or problems here in the comment section below.